Analysis of the Inequality of the Curve and the Straight Line (Extract)

Introduction

During the geometric developments carried out in his the first book “The Inequality of the Curve and the Straight Line,” a trend to a given relationship, or pattern is discovered by means of a research based on the “trial and error” method. In determining such pattern by means of an iterative method of geometric construction, a triangle of surface equal to the surface of a given circumference was obtained.

If DABC is an isosceles triangle with a height of 2d and a base equal to the perimeter of the circumference of diameter d, its surface will be equal to the surface of the circumference of diameter 2d.

Following the development of this pattern, equalities could be established for perimeters of circumference with circumferences having a smaller diameter and equalities of square perimeters with squares having a smaller side. Also, equalities of circle surface areas with circles of a smaller diameter and equalities of sphere volumes with volumes of spheres of a smaller diameter.

In order to simplify this study, I built 38 models or explicative shapes for exhibitions and conventions at several universities in Mexico, Brazil, Argentina and Chile, among others.

Virtual video of explicative models

EQUALITY OF CIRCUMFERENCE PERIMETERS

The perimeter of a circumference is equal to the sum of the perimeters of N tangent circumferences aligned along its diameter.

DEMONSTRATION:

Let d₁, d₂, ..., d_n, be the diameters of the N circumferences, and let P₁, P₂, ..... , P_n be the perimeters of such circumferences. Then, perimeter P of the circumference of diameter d is:

P = πd

= π(d₁ + d₂ + ..... + d_n)

= πd₁ + πd₂ + ..... + πd_n

= P₁ + P₂ + .... + P_n

NOTE: A Simple Example

The minimum expression for the circumference diameter before arriving to the unit is 2 units, so the perimeter of the circumference is 2π. We can draw 2 tangent circumferences with a diameter of 1 U and aligned along its diameter, each with a perimeter equal to π.

EQUALITY OF SQUARE PERIMETERS

The sum of the perimeters of any "n" squares is equal to the perimeter of a square whose side "s" is equal to the sum of the "n" squares.

Illustration

for n = 4

Demonstration

Let s₁, s₂, s₃, ... s_n be the sides of the "n" squares.

Let P₁, P₂, P₃, ... P_n be the perimeters of such squares.

Then, the perimeter P of the square with side s is:

P = 4s

P = 4(s₁ + s₂ + s₃  + ... + s_n)

P = 4s₁ + 4s₂ + 4s₃ + ... + 4s_n

P = P₁ + P₂ + P₃ + ... + P_n

CONCLUSION 1

With the equality of circumference perimeters and the equality of square perimeters, I have arrived to the following conclusion, which was published on page 214 of the Book “Abstracts – of the XVII Latin American Meeting on Educational Mathematics”  (RELME17, by its Spanish acronym), Catholic University, Santiago, Chile – July 2003.

Excerpt

*****************************************************************

PN0505

INEQUALITY OF THE CURVE AND THE STRAIGHT LINE ANALYSIS

Structural foundations for the extension of the systems of measurement

Walter Enrique Meyer Vergara

curiosidadesgeometricas@gmail.com

Field of Research

Educational Level: All levels

“The mathematical relation of the curve and the straight line is equal. Thus, it is also equal for squares and circumferences, as well as cubes and spheres.”  In simple terms, we could say that the same way we operate with squares, we may do it with circumferences and the same way we operate with cubes, we operate with spheres in an exact way.

The scheme formed by the circumference and the square formed by its tangents go from zero to infinite. This also applies to spheres and the cube formed by their tangential planes. Thus, we may assert that the definition we have of a straight line saying that it is a curve of infinite radius, is false.

La devise of a method to measure EXACTLY the whole circular and spherical world, in accordance with the “future times.”

****************************************************************

Note:  When I refer to the word "EXACT," it is in geometry and not as for measurement systems.

***************************************************************************************

CONCLUSION 2

Calculation of Circumference Arc Perimeters

P = Perimeter

R = Radius
O = Circumferential Unit
n = number of circunferentials
U = Unit

 

The following illustrations correspond to the second part of the first book, which are practical conclusions made from the perimeter equality study. 

UNIT OF MEASUREMENT

We have known many “Units of Length” a través del tiempo, so we will analyze only the two main linear units that we have nowadays.

Fundamental Units of Length, called “METER”

(1 meter = 100 centimeters = 1,000 millimeters……etc.)

Customary Unit of Length, called “YARD”

(1 yard = 3 feet = 36 inches……etc.)

Now, we have been able to see in this study that with the “Equality of Perimeters” we may calculate circumference perimeters, circle areas, sphere volumes, etc., de same way we do it with linear units for cube volumes, square areas, etc. So I would like to set forth the idea of extending the aforementioned linear longitude patterns, so as to obtain also a circumferential measure pattern, circular and spherical, that allows us to calculate directly all the “Spherical Universe.”

If we take any of this pattern units (meters or yards) as the diameter of a circumference, we may determine each diameter as:

Diametral Meter or Diametral Yard

And the perimeter of each circumference may be called:

CIRCUMFERENTIAL METER OR CIRCUMFERENTIAL YARD

In order to calculate perimeters, we have that:

1 circumferential meter……… (Cm)   = 10 circumferential decimeters (Cdm)

1 circumference centimeter … (Ccm) = 10 circumferential millimeters (Cmm)

etc.

In order to calculate surfaces, we have that:

1 circular meter……….. (cm)            = 10 square circular decimeters (cdm)²

1 circular centimeter…. (ccm)           = 10 square circular millimeters (cmm)²

1 circular millimeter….. (cmm)           = 10 square circular tenths of a millimeter

etc.

In order to calculate volumes, we have that:

1 spherical meter……. (Sm)     =  10 cubic spherical decimeters (Sdm)³

                                               = 100 cubic spherical centimeters (Scm)³

1 spherical centimeter (Scm)   =   10 cubic spherical millimeters (Smm)³

If we observe page 51 closely, what I have called “General System, we realize that the model formed with the spheres and cubes given by the tangents (tangential planes) of the spheres (linear system), operate the same way as for several calculations of volume, area, etc.

Note:

We have this system configuration, the way it operates and its exactitude in multiple physical phenomena.

For example:


a) Sound reflection propagating in a straight line, in circular waves similar to those produced when a stone falls into the water, although sound waves propagate in all directions (spherical) and not just horizontally (explosion case).

     b)The electromagnetic wave propagation emitted by a point source.
     c)   Light emission.    

 

CIRCUMFERENTIAL METER

    This first “Circumferential Meter” has been built in white melamine and its perimeter highlighted with a copper sheet. The linear meter is made of millimeter steel and circumferential decimeters are copper circles. The first 10 Ccm are also highlighted with stainless steel discs.

 Note: The equal sign (=) of this picture means: Equal relation

 

As we can see, the linear meter is divided into 10 decimeters, but the perimeter (distance around the 10 circumferences) is equal to the perimeter of the circumferential meter.  If the meter is divided into 100 linear centimeters, the perimeters of the 100 circumferences is equal to the perimeter of the circumferential meter,... , etc. This is also true for other measurement systems, for example the yard or the new inch, etc.   This will be explained later when we advance with the study.

NEW ANALYSIS OF MEASUREMENT SYSTEM

  

FIRST RULE TO MEASURE STRAIGHT LINES AND CIRCUMFERENCE PERIMETERS

Analysis of Circumferential geometric measurement Units 

 

Note

Measurements of length for:

1 Nautical Mile = 1,852 m. This unit corresponds to the mean distance between two points of the terrestrial surface of equal longitude and whose latitude differs in 1 minute. (year 1929)

a.- 1 light-year measured with geometric miles.

1 light-year = 9,461 trillion Km (9,461,000,000,000)

“Length unit equal to the distance that light travels in vacuum in one year.”

1 light year = 5,160 trillion “geometric miles” (5,160,175,018,000), measurement obtained by multiplying 5,160…x 1,8334… = 9,46… trillion Km.

1 light year = 29,722 trillion cKm (29,722,608,103,680), value obtained by multiplying  5,160.175.018.000 x 5,76 cKm.

 

Model of exhibition 

 

 

Model representative of the linear and circumferential meter

I hereby present the first gauge (meter stick), extending the idea of the first meter, which measures linear measurements with the new Chilean inch and linear measurements with the metric system, put forward on pages 104-105 and 106 of the first book and on page 3 of the Study of the First Chilean Yard.

            When we measure the diameter of an axis, this meter stick directly gives us the circumference perimeter, thus making it easier for the operator of a machine tool to calculate the cutting speed.  Such speed varies according to the diameter and consequently the perimeter of the workpiece to be milled and the specification given by the manufacturer of each tool.

Examples of Measuring Instruments, Application and Use of the System 

Application of the circumferential meter and inch patterns to the meter gauge and micrometer for its use in the development of milling processes.

If we measure the diameter of an axis, we directly obtain the perimeter, making it easier for a machine tool operator to calculate the cutting speed.

S = peripheral cutting speed

         in meters per minute

S  = pi Í DN  /1000

N = number of rotations per

       minute of the tooling (spindle and boring head of a milling machine)

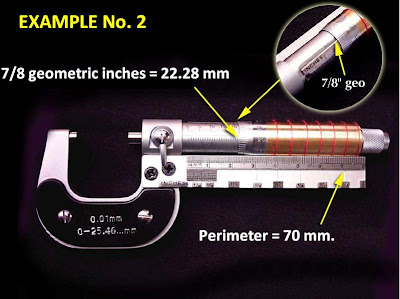
With this   micrometer we may measure up to 1 geometric inch; we may measure centimeters, millimeters, tenths and hundredths of a mm; we may measure circumferential perimeters, etc.

Calculation of the surface area de circle with circles of a smaller diameter with the new circular system

We present the traditional method of calculation to obtain the formula for the surface area of a circle, which is equal to pi x r2  (measured with square units) and to be able to compare it to the new circular system proposed, where we measure the surface of a circle with circles of smaller diameter using the formula  (2r)2 circles.  We concluded that the simplicity of this formula could help mathematics to be more didactic, a recurrent subject in conventions on mathematics.

CALCULATION OF VOLUMES WITH THE NEW SPHERICAL MEASUREMENT SYSTEM

STUDY OF THE METRIC UNIT CALLED LITER

We know that 1 liter is equivalent to 1,000 cm³ and serves indistinctly for liquids and aggregates.

We have represented it with a cubic acrylic model with sides measuring 10 cm, containing 1,000 small 1cm³-wooden cubes (A).  It is evident that if we keep on increasing such volume (200 liters, 50,000 liters, etc.) we have difficulty of transport, material resistance, etc. Thus, we were forced to make cylinders (rounded base drums, elliptical base drums, or simply spheres).

If we make a cylinder, we have exactitude in the surface of the circle (surface = p r²) and in its height (h = 1,000/p r²).  See graduated jar (B).

Now then, with our calculation system, we may obtain exactitude of the surface area of the cylinder circle, by calculating it with ccm (circular centimeters) and inexactitude in its height. I have represented this with a 2-liter graduated jar (C) (Page 107) having 8 cm. in diameter, so the surface area of the circle is equal to 64 ccm.

With the 64 circular centimeters I made cylinders of 39.788735... cm. in height having a volume of 31.25 cm³ each (D). The model with 64 aluminum cylinders is accompanied by an aluminum cylinder and a glass tube (E), which contains 59.6831... 1 cm-diameter spheres, calculation obtained from the cylinder to sphere ratio of 2/3. This ratio obtained by Archimedes, is shown in model (F), which is quite didactic and also presents the “Cylinder and Sphere” equality of.

(F) Cylinder of diameter D and height D = 1 ½ sphere of diameter D

The new sequence of models (G-H, page 108) show, this time with spheres, full exactitude for these calculations, naturally for a new liter that has a ratio equal to p/6 with regards to our cubic liter.

We put forward again an acrylic cubic model (G) measuring 10 cm in side, but this time with 1,000 Scm (spherical centimeters) that are exactly equivalent to a 10 cm-diameter sphere (spherical flask H).

Then, with a 5 cm diameter graduated jar (I) we calculate the surface of the circle with ccm (circular centimeters) equal to 25 ccm. With each circular centimeter we make tubes that contain exactly 40 1 cm-diameter spheres. We multiply these spheres by 2/3 and we obtain cylinders of 1ccm by 26.666... cm of height (J).

With the 25 cylinders (K), we obtain exactly the volume of the graduated jar of diameter 5 and the flask of diameter 10 cm. (I and H).

Note:

The height of the aluminum cylinders, equal to 26.666...cm is in practice 26 cm (26 units) + exactly 2/3 of 1cm.

==================================================

Model representing physically the equality of a 4-unit-diameter sphere volume that is equal to a cube of 1-unit-diameter spheres (that is, a 64 unit cube).

After this excerpt of the first book "Analysis of the Inequality of the Curve and the Straight Line”, we will now analyze the Study of Circle and Square Surfaces that contain a more thorough analysis of the circular and spherical curve analysis.

===========================================================